Next Нерегулярный Сумдоку 9х9 5
 Нерегулярный Сумдоку 9х9 2 Previous
Нерегулярный Сумдоку 9х9 2 Previous
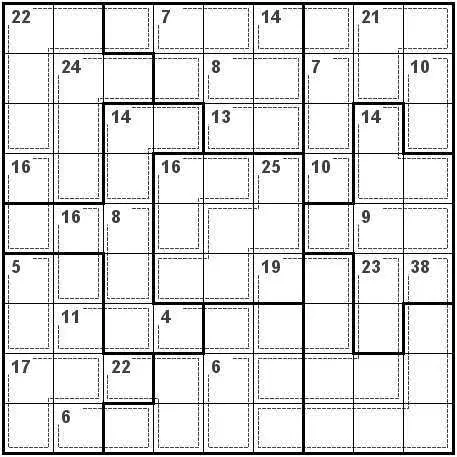
Mathematical Sumoku online is created by the authors of the site crossword.nalench.com as a sudoku variant of the same size: and consists of traditional 9x9 squares. However, despite the differences, it retains the basic rules that define them: you must put numbers from 1 to 9, one in each field and without repeats, and there is only one possible solution.
Being so small, instead of showing some figures, from which others are deduced, as in a traditional sudoku, another type of hint is indicated for its solution, namely:
To the right of each row and below each column is displayed a number corresponding to the total of three numbers in the corresponding row or column.
For example: if the number 16 appears to the right of the first row, it means that the sum of the numbers of 3 fields in that row should result in 16. The same rule applies to the other rows as well as to the 3 columns, each field at the top indicates whether the number found is prime, even, or odd. All fields with prime numbers will always be listed as such. This is exceptional, that is, in other fields where odd or even are specified, all cousins and sisters may be excluded.
For example: suppose 2. This is a prime number, but also a pair. A field that matches this will always point to a cousin and never connect, even if it is. Thus, we know that the other fields marked as pairs will never have 2. The same logic applies to other primes.
It is clear that if the mini-sudoku is completely empty from the start, without any numbers as a clue, the only way left to solve it is to consider the possible numbers that can be indicated in each window, or candidates, as they are commonly called in the world of sumoku. . And from there, analyzing the rows and columns with their totals, try to discard the ones that are impossible until you find the ones that are.
The possible candidates, according to the prompt given in each box, and taking into account what is explained in the “What is it?” Section, are as follows:
For fields given as prime numbers: 2, 3, 5, and 7 (only cousins between 1 and 9 are possible). For marked as an even number: 4, 6 and 8 (2 is discarded because it is a prime number). For designated as odd: 1 and 9 (3, 5 and 7 are odd, but also siblings, and remember that whenever the number is prime, it is specified as such with full priority, allowing them to be dropped from other fields).
Boxes with fewer candidates are always referred to as odd: only 2. Therefore, statistically, they are more likely to be detected easier and faster. Having 2 candidates also means that there will always be only 2 boxes in each sumdock marked with an odd number. If you find one by dropping, you will also find another. That is, if you know that only one cell can be placed in one cell, it must go to the other in the other 9. And vice versa.
The next recommended step is to first analyze the rows and columns whose sum is the smallest value, since there will be statistically fewer possible combinations of the 3 numbers that are summed to that value. We have more opportunities and opportunities to find out some number or to refuse any candidate
Then all is to analyze the candidates we have in each row and column, and see what the possibilities are to reach the sum, or, conversely, to see that it is mathematically impossible to reject the candidates to be able to decipher the numbers.

 Нерегулярный Сумдоку 9х9 2 Previous
Нерегулярный Сумдоку 9х9 2 PreviousIrregular Sumdoku 9x9 3 - what's the puzzle?
Mathematical Sumoku online is created by the authors of the site crossword.nalench.com as a sudoku variant of the same size: and consists of traditional 9x9 squares. However, despite the differences, it retains the basic rules that define them: you must put numbers from 1 to 9, one in each field and without repeats, and there is only one possible solution.
Being so small, instead of showing some figures, from which others are deduced, as in a traditional sudoku, another type of hint is indicated for its solution, namely:
To the right of each row and below each column is displayed a number corresponding to the total of three numbers in the corresponding row or column.
For example: if the number 16 appears to the right of the first row, it means that the sum of the numbers of 3 fields in that row should result in 16. The same rule applies to the other rows as well as to the 3 columns, each field at the top indicates whether the number found is prime, even, or odd. All fields with prime numbers will always be listed as such. This is exceptional, that is, in other fields where odd or even are specified, all cousins and sisters may be excluded.
For example: suppose 2. This is a prime number, but also a pair. A field that matches this will always point to a cousin and never connect, even if it is. Thus, we know that the other fields marked as pairs will never have 2. The same logic applies to other primes.
How does Sumdok decide?
It is clear that if the mini-sudoku is completely empty from the start, without any numbers as a clue, the only way left to solve it is to consider the possible numbers that can be indicated in each window, or candidates, as they are commonly called in the world of sumoku. . And from there, analyzing the rows and columns with their totals, try to discard the ones that are impossible until you find the ones that are.
The possible candidates, according to the prompt given in each box, and taking into account what is explained in the “What is it?” Section, are as follows:
For fields given as prime numbers: 2, 3, 5, and 7 (only cousins between 1 and 9 are possible). For marked as an even number: 4, 6 and 8 (2 is discarded because it is a prime number). For designated as odd: 1 and 9 (3, 5 and 7 are odd, but also siblings, and remember that whenever the number is prime, it is specified as such with full priority, allowing them to be dropped from other fields).
Once this is clarified, two questions emerge:
Boxes with fewer candidates are always referred to as odd: only 2. Therefore, statistically, they are more likely to be detected easier and faster. Having 2 candidates also means that there will always be only 2 boxes in each sumdock marked with an odd number. If you find one by dropping, you will also find another. That is, if you know that only one cell can be placed in one cell, it must go to the other in the other 9. And vice versa.
The next recommended step is to first analyze the rows and columns whose sum is the smallest value, since there will be statistically fewer possible combinations of the 3 numbers that are summed to that value. We have more opportunities and opportunities to find out some number or to refuse any candidate
Then all is to analyze the candidates we have in each row and column, and see what the possibilities are to reach the sum, or, conversely, to see that it is mathematically impossible to reject the candidates to be able to decipher the numbers.

Answer to Irregular Sumdoku 9x9 3
Comments:
Be the first to comment on the crossword puzzle!
New crossword puzzle comments:
Петро Полюхович © 2011-2024
Join us if you are a true crossword puzzler!


 Login
Login Crosswords
Crosswords Conundrums
Conundrums Sudoku
Sudoku English
English Turkish
Turkish About
About